| 书目名称 | Dynamical Systems, Bifurcation Analysis and Applications | | 副标题 | Penang, Malaysia, Au | | 编辑 | Mohd Hafiz Mohd,Norazrizal Aswad Abdul Rahman,Yaza | | 视频video | http://file.papertrans.cn/284/283901/283901.mp4 | | 概述 | Offers latest contents and developments in dynamical systems and bifurcation analysis.Maximizes reader insights into selected topics by active and well-known researchers.Incorporates a wealth of resea | | 丛书名称 | Springer Proceedings in Mathematics & Statistics | | 图书封面 |  | | 描述 | This book is the result of Southeast Asian Mathematical Society (SEAMS) School 2018 on Dynamical Systems and Bifurcation Analysis (DySBA). It addresses the latest developments in the field of dynamical systems, and highlights the importance of numerical continuation studies in tracking both stable and unstable steady states and bifurcation points to gain better understanding of the dynamics of the systems. .The SEAMS School 2018 on DySBA was held in Penang from 6th to 13th August at the School of Mathematical Sciences, Universiti Sains Malaysia.The SEAMS Schools are part of series of intensive study programs that aim to provide opportunities for an advanced learning experience in mathematics via planned lectures, contributed talks, and hands-on workshop..This book will appeal to those postgraduates, lecturers and researchers working in the field of dynamical systems and their applications. Senior undergraduates in Mathematics will also find it useful.. | | 出版日期 | Conference proceedings 2019 | | 关键词 | dynamical systems; bifurcation; population dynamics; disease resistance; dispersal models; riddled basin; | | 版次 | 1 | | doi | https://doi.org/10.1007/978-981-32-9832-3 | | isbn_softcover | 978-981-32-9834-7 | | isbn_ebook | 978-981-32-9832-3Series ISSN 2194-1009 Series E-ISSN 2194-1017 | | issn_series | 2194-1009 | | copyright | Springer Nature Singapore Pte Ltd. 2019 |
The information of publication is updating

书目名称Dynamical Systems, Bifurcation Analysis and Applications影响因子(影响力)

书目名称Dynamical Systems, Bifurcation Analysis and Applications影响因子(影响力)学科排名

书目名称Dynamical Systems, Bifurcation Analysis and Applications网络公开度
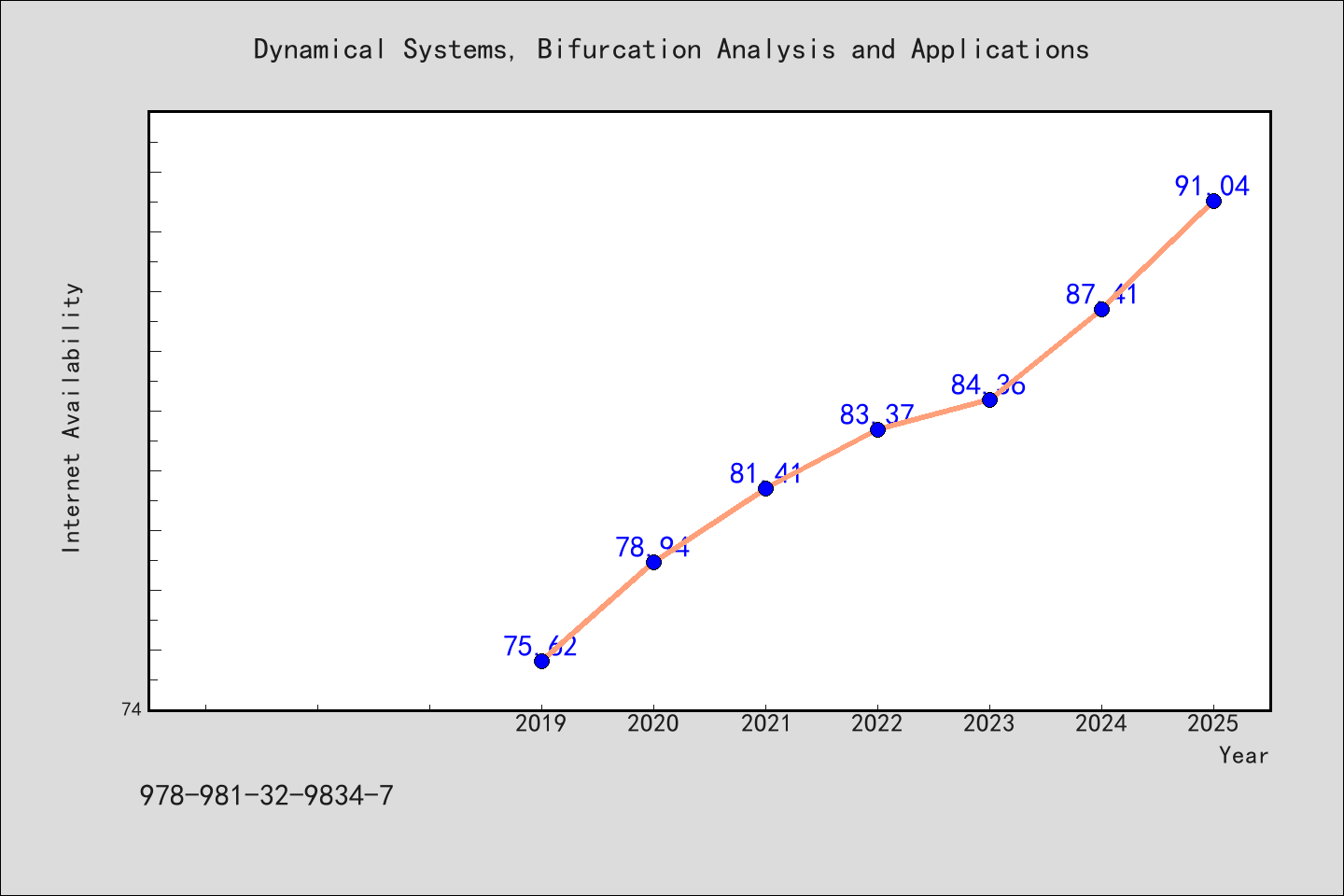
书目名称Dynamical Systems, Bifurcation Analysis and Applications网络公开度学科排名
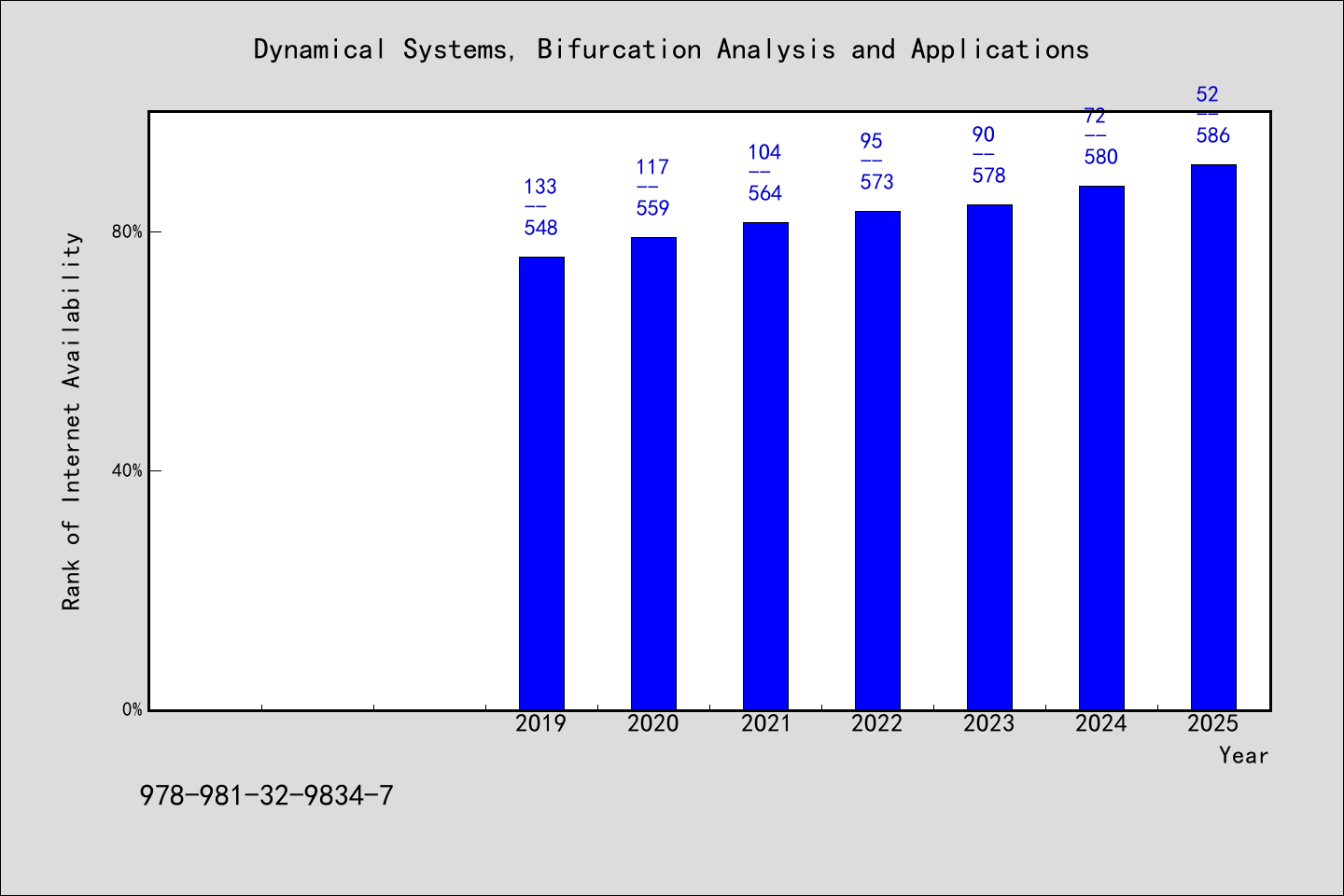
书目名称Dynamical Systems, Bifurcation Analysis and Applications被引频次

书目名称Dynamical Systems, Bifurcation Analysis and Applications被引频次学科排名

书目名称Dynamical Systems, Bifurcation Analysis and Applications年度引用

书目名称Dynamical Systems, Bifurcation Analysis and Applications年度引用学科排名

书目名称Dynamical Systems, Bifurcation Analysis and Applications读者反馈

书目名称Dynamical Systems, Bifurcation Analysis and Applications读者反馈学科排名

|
|
|
 |Archiver|手机版|小黑屋|
派博传思国际
( 京公网安备110108008328)
GMT+8, 2025-8-21 21:19
|Archiver|手机版|小黑屋|
派博传思国际
( 京公网安备110108008328)
GMT+8, 2025-8-21 21:19


