| 书目名称 | Distance Expanding Random Mappings, Thermodynamical Formalism, Gibbs Measures and Fractal Geometry | | 编辑 | Volker Mayer,Mariusz Urbanski,Bartlomiej Skorulski | | 视频video | http://file.papertrans.cn/282/281664/281664.mp4 | | 概述 | Contains new results.Complete treatment of the topic.Originality of the topic.Includes supplementary material: | | 丛书名称 | Lecture Notes in Mathematics | | 图书封面 |  | | 描述 | .The theory of random dynamical systems originated from stochastic.differential equations. It is intended to provide a framework and.techniques to describe and analyze the evolution of dynamical.systems when the input and output data are known only approximately, according to some probability distribution. The development of this field, in both the theory and applications, has gone in many directions. In this manuscript we introduce measurable expanding random dynamical systems, develop the thermodynamical formalism and establish, in particular, the exponential decay of correlations and analyticity of the expected pressure although the spectral gap property does not hold. This theory is then used to investigate fractal properties of conformal random systems. We prove a Bowen’s formula and develop the multifractal formalism of the Gibbs states. Depending on the behavior of the Birkhoff sums of the pressure function we arrive at a natural classification of the systems into two classes: quasi-deterministic systems, which share many.properties of deterministic ones; and essentially random systems, which are rather generic and never bi-Lipschitz equivalent to deterministic systems. We s | | 出版日期 | Book 2011 | | 关键词 | 37-XX; Hausdorff dimension; multifractal spectrum; random dynamical systems; thermodynamical formalism | | 版次 | 1 | | doi | https://doi.org/10.1007/978-3-642-23650-1 | | isbn_softcover | 978-3-642-23649-5 | | isbn_ebook | 978-3-642-23650-1Series ISSN 0075-8434 Series E-ISSN 1617-9692 | | issn_series | 0075-8434 | | copyright | Springer-Verlag Berlin Heidelberg 2011 |
The information of publication is updating

书目名称Distance Expanding Random Mappings, Thermodynamical Formalism, Gibbs Measures and Fractal Geometry影响因子(影响力)

书目名称Distance Expanding Random Mappings, Thermodynamical Formalism, Gibbs Measures and Fractal Geometry影响因子(影响力)学科排名

书目名称Distance Expanding Random Mappings, Thermodynamical Formalism, Gibbs Measures and Fractal Geometry网络公开度
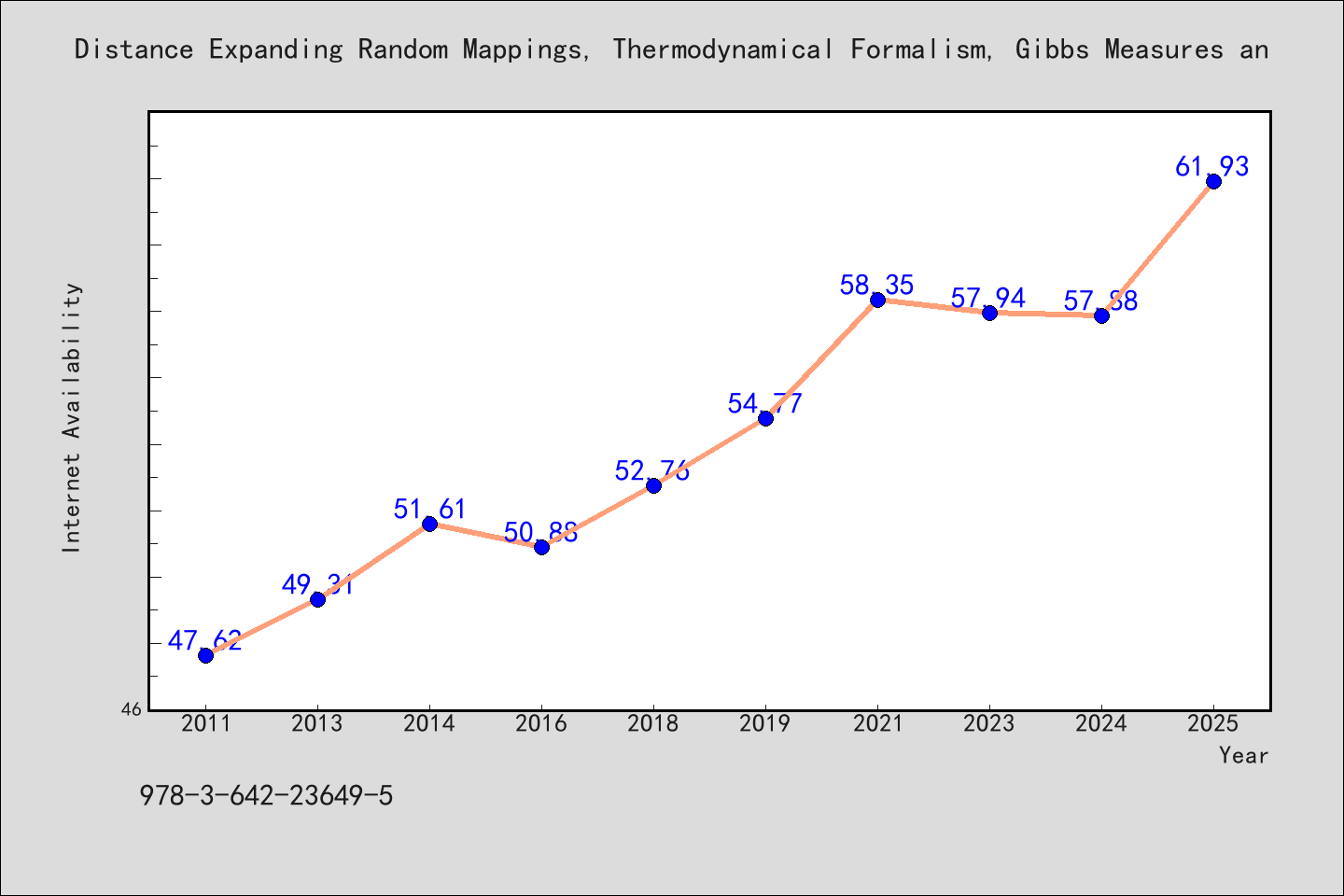
书目名称Distance Expanding Random Mappings, Thermodynamical Formalism, Gibbs Measures and Fractal Geometry网络公开度学科排名
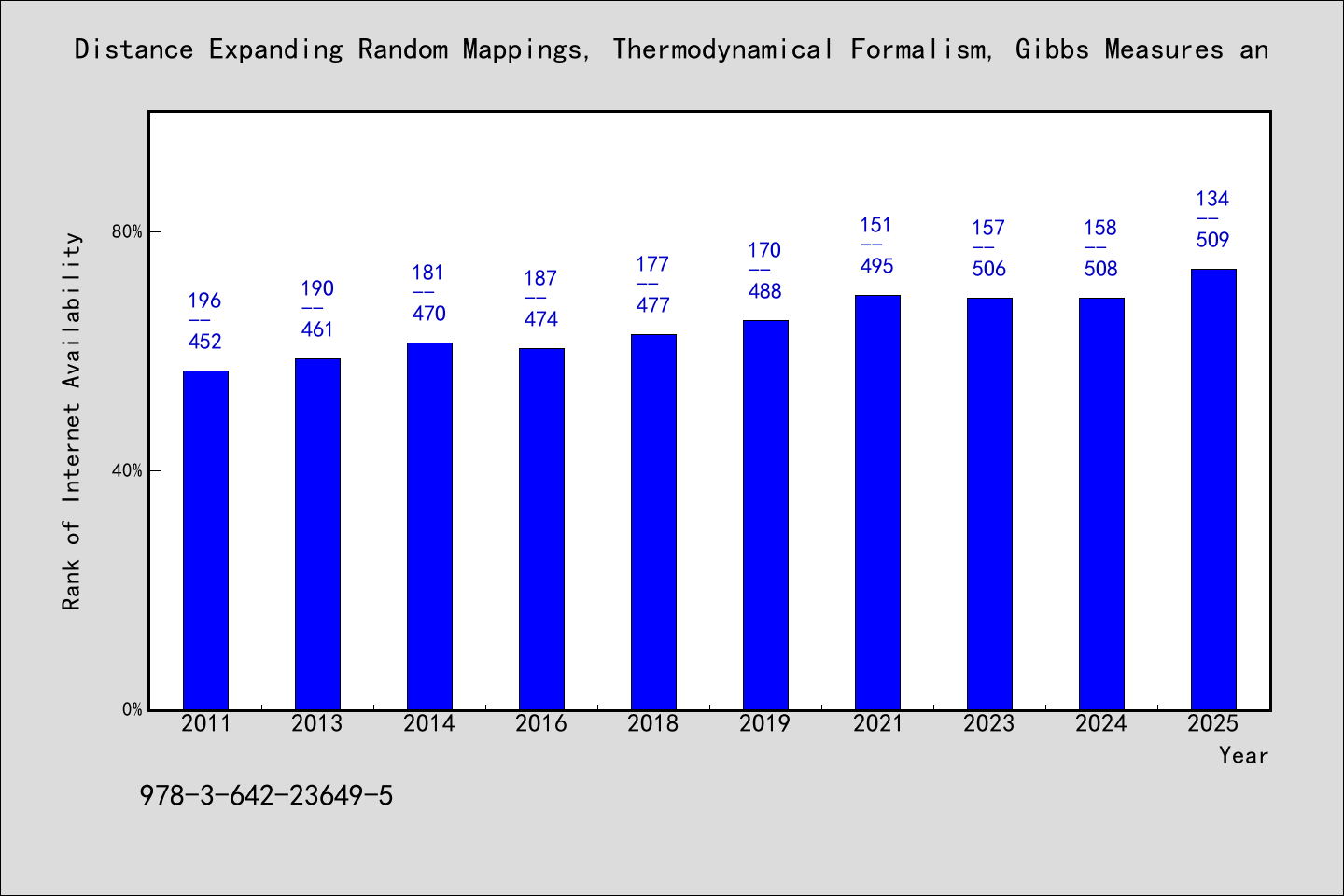
书目名称Distance Expanding Random Mappings, Thermodynamical Formalism, Gibbs Measures and Fractal Geometry被引频次

书目名称Distance Expanding Random Mappings, Thermodynamical Formalism, Gibbs Measures and Fractal Geometry被引频次学科排名

书目名称Distance Expanding Random Mappings, Thermodynamical Formalism, Gibbs Measures and Fractal Geometry年度引用

书目名称Distance Expanding Random Mappings, Thermodynamical Formalism, Gibbs Measures and Fractal Geometry年度引用学科排名

书目名称Distance Expanding Random Mappings, Thermodynamical Formalism, Gibbs Measures and Fractal Geometry读者反馈

书目名称Distance Expanding Random Mappings, Thermodynamical Formalism, Gibbs Measures and Fractal Geometry读者反馈学科排名

|
|
|
 |Archiver|手机版|小黑屋|
派博传思国际
( 京公网安备110108008328)
GMT+8, 2025-8-21 13:48
|Archiver|手机版|小黑屋|
派博传思国际
( 京公网安备110108008328)
GMT+8, 2025-8-21 13:48


