| 书目名称 | The Non-Euclidean, Hyperbolic Plane | | 副标题 | Its Structure and Co | | 编辑 | Paul Kelly,Gordon Matthews | | 视频video | | | 丛书名称 | Universitext | | 图书封面 |  | | 描述 | The discovery of hyperbolic geometry, and the subsequent proof that this geometry is just as logical as Euclid‘s, had a profound in fluence on man‘s understanding of mathematics and the relation of mathematical geometry to the physical world. It is now possible, due in large part to axioms devised by George Birkhoff, to give an accurate, elementary development of hyperbolic plane geometry. Also, using the Poincare model and inversive geometry, the equiconsistency of hyperbolic plane geometry and euclidean plane geometry can be proved without the use of any advanced mathematics. These two facts provided both the motivation and the two central themes of the present work. Basic hyperbolic plane geometry, and the proof of its equal footing with euclidean plane geometry, is presented here in terms acces sible to anyone with a good background in high school mathematics. The development, however, is especially directed to college students who may become secondary teachers. For that reason, the treatment is de signed to emphasize those aspects of hyperbolic plane geometry which contribute to the skills, knowledge, and insights needed to teach eucli dean geometry with some mastery. | | 出版日期 | Textbook 1981 | | 关键词 | Hyperbolische Geometrie; Plane; congruence; construction; function; geometry; hyperbolic geometry; knowledg | | 版次 | 1 | | doi | https://doi.org/10.1007/978-1-4613-8125-9 | | isbn_softcover | 978-0-387-90552-5 | | isbn_ebook | 978-1-4613-8125-9Series ISSN 0172-5939 Series E-ISSN 2191-6675 | | issn_series | 0172-5939 | | copyright | Springer-Verlag New York, Inc. 1981 |
The information of publication is updating
书目名称The Non-Euclidean, Hyperbolic Plane影响因子(影响力)
书目名称The Non-Euclidean, Hyperbolic Plane影响因子(影响力)学科排名
书目名称The Non-Euclidean, Hyperbolic Plane网络公开度
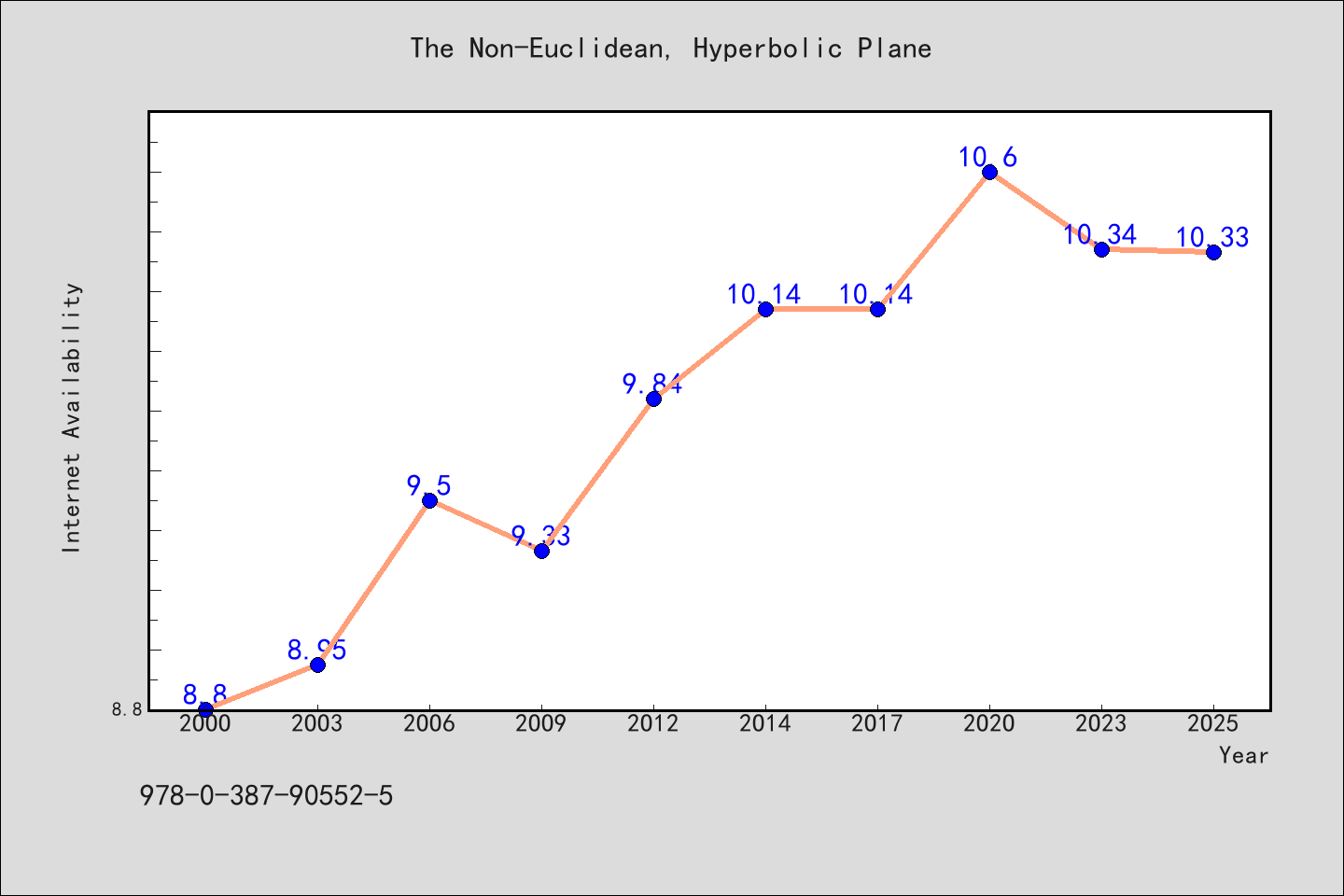
书目名称The Non-Euclidean, Hyperbolic Plane网络公开度学科排名
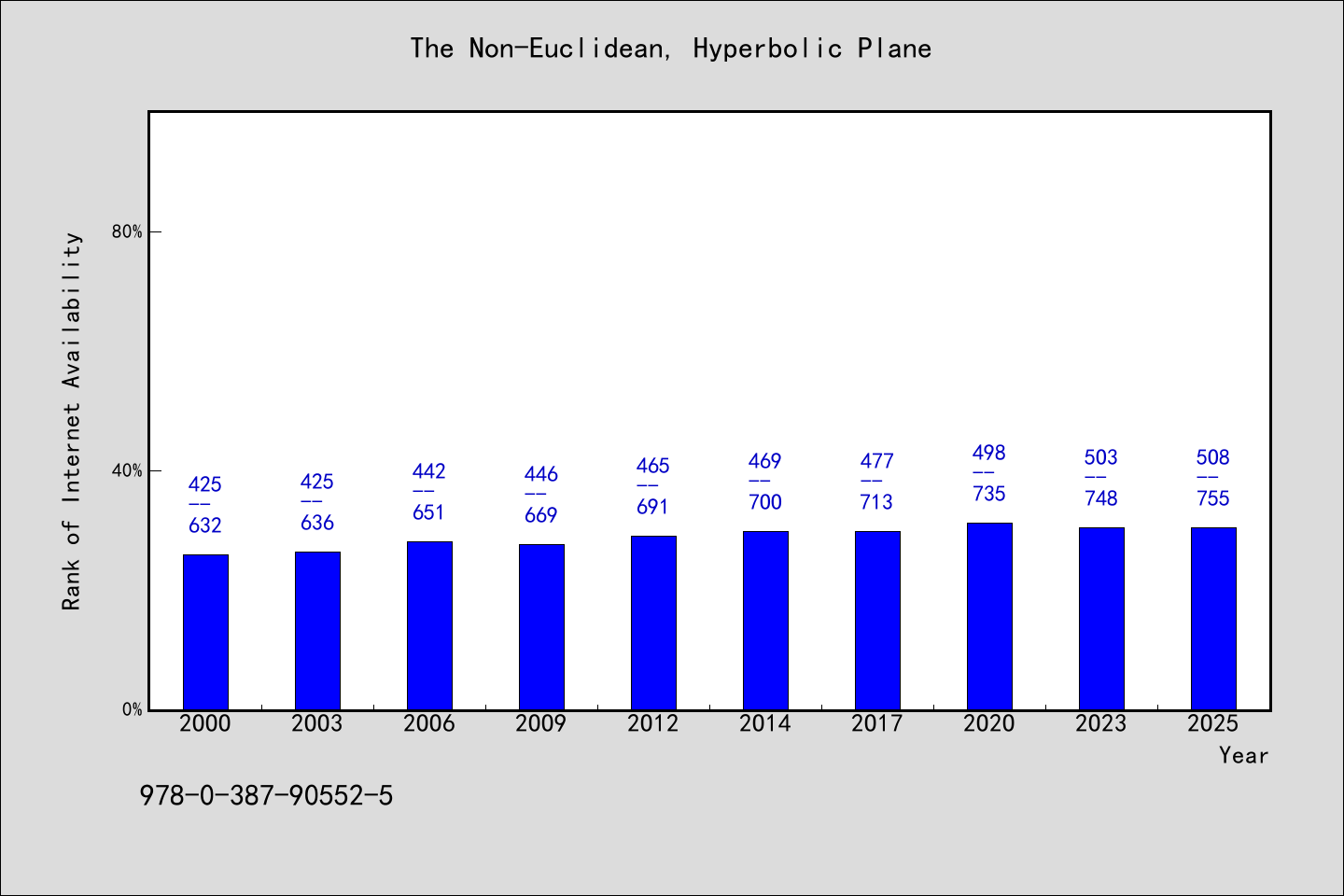
书目名称The Non-Euclidean, Hyperbolic Plane被引频次
书目名称The Non-Euclidean, Hyperbolic Plane被引频次学科排名
书目名称The Non-Euclidean, Hyperbolic Plane年度引用
书目名称The Non-Euclidean, Hyperbolic Plane年度引用学科排名
书目名称The Non-Euclidean, Hyperbolic Plane读者反馈
书目名称The Non-Euclidean, Hyperbolic Plane读者反馈学科排名
|
|
|
发表于 2025-3-21 19:55:27
