| 书目名称 | Random Walk, Brownian Motion, and Martingales | | 编辑 | Rabi Bhattacharya,Edward C. Waymire | | 视频video | | | 概述 | Offers an accessible introduction to the rigorous study of stochastic processes.Builds from simple examples to formal proofs, illuminating key ideas and computations.Showcases a selection of important | | 丛书名称 | Graduate Texts in Mathematics | | 图书封面 |  | | 描述 | .This textbook offers an approachable introduction to stochastic processes that explores the four pillars of random walk, branching processes, Brownian motion, and martingales. Building from simple examples, the authors focus on developing context and intuition before formalizing the theory of each topic. This inviting approach illuminates the key ideas and computations in the proofs, forming an ideal basis for further study...Consisting of many short chapters, the book begins with a comprehensive account of the simple random walk in one dimension. From here, different paths may be chosen according to interest. Themes span Poisson processes, branching processes, the Kolmogorov–Chentsov theorem, martingales, renewal theory, and Brownian motion. Special topics follow, showcasing a selection of important contemporary applications, including mathematical finance, optimal stopping, ruin theory, branching random walk, and equations of fluids. Engaging exercises accompany the theorythroughout...Random Walk, Brownian Motion, and Martingales. is an ideal introduction to the rigorous study of stochastic processes. Students and instructors alike will appreciate the accessible, example-driven | | 出版日期 | Textbook 2021 | | 关键词 | Stochastic processes textbook; Random walk mathematics; Branching processes mathematics; Martingales ma | | 版次 | 1 | | doi | https://doi.org/10.1007/978-3-030-78939-8 | | isbn_ebook | 978-3-030-78939-8Series ISSN 0072-5285 Series E-ISSN 2197-5612 | | issn_series | 0072-5285 | | copyright | Springer Nature Switzerland AG 2021 |
The information of publication is updating
书目名称Random Walk, Brownian Motion, and Martingales影响因子(影响力)
书目名称Random Walk, Brownian Motion, and Martingales影响因子(影响力)学科排名
书目名称Random Walk, Brownian Motion, and Martingales网络公开度
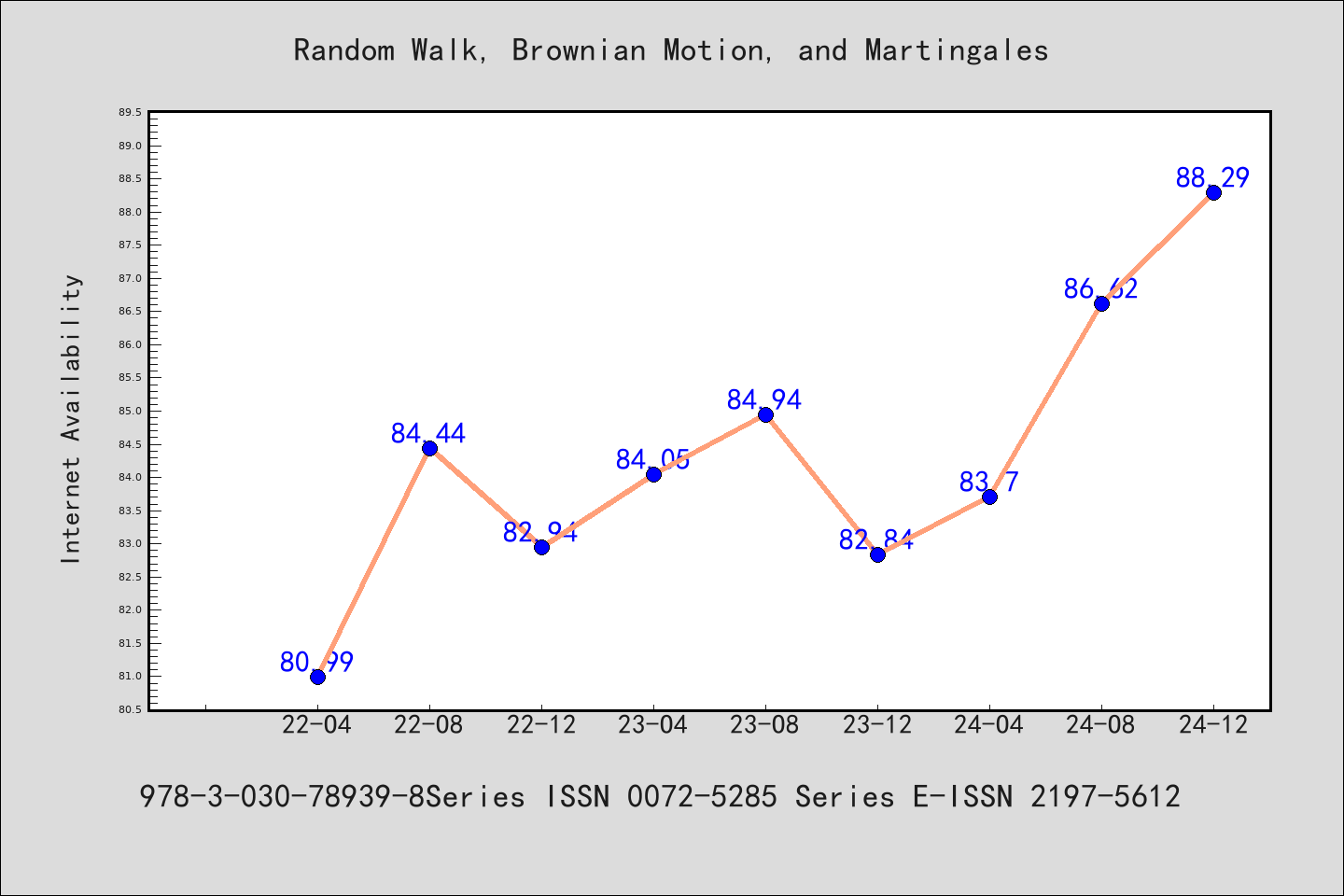
书目名称Random Walk, Brownian Motion, and Martingales网络公开度学科排名
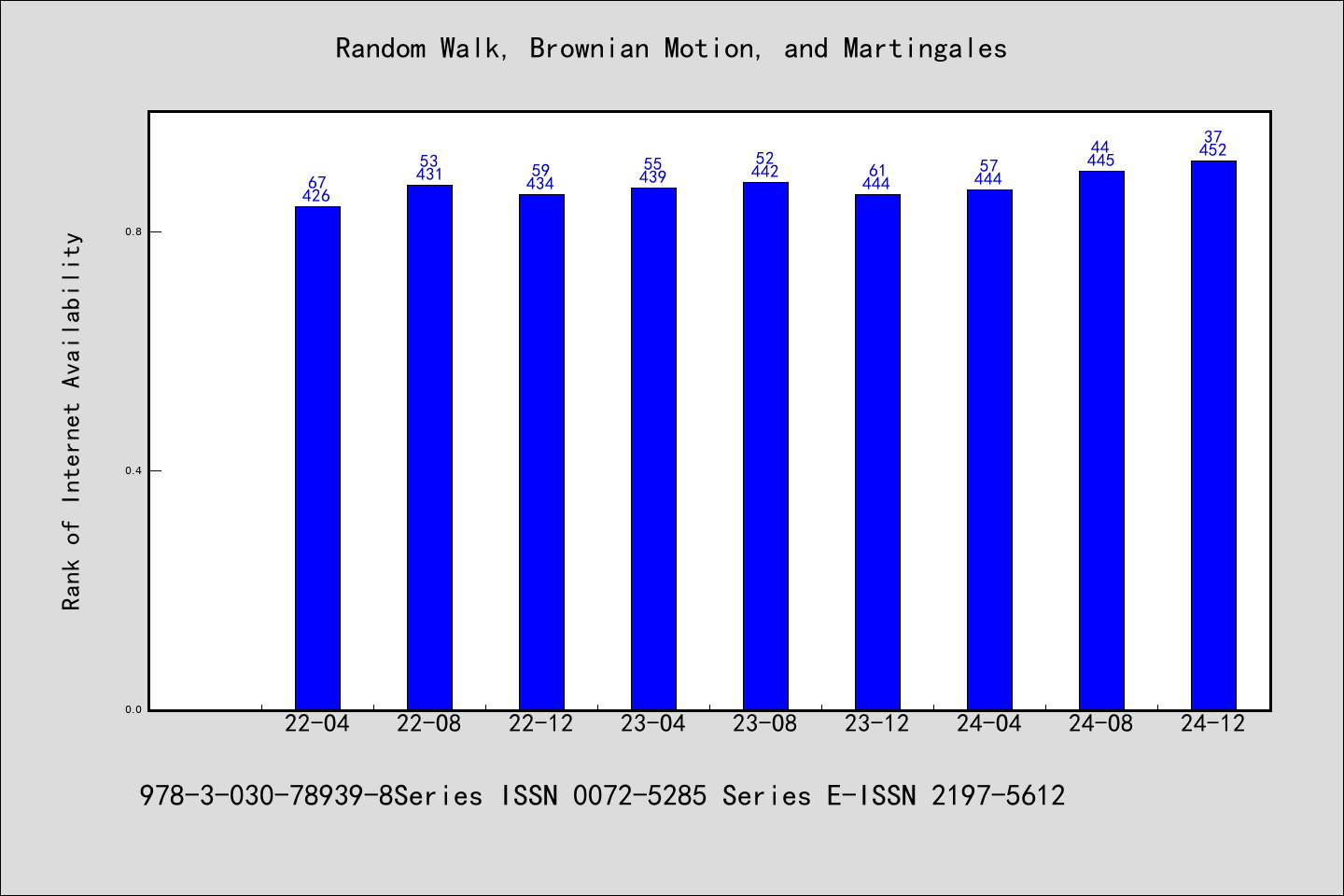
书目名称Random Walk, Brownian Motion, and Martingales被引频次
书目名称Random Walk, Brownian Motion, and Martingales被引频次学科排名
书目名称Random Walk, Brownian Motion, and Martingales年度引用
书目名称Random Walk, Brownian Motion, and Martingales年度引用学科排名
书目名称Random Walk, Brownian Motion, and Martingales读者反馈
书目名称Random Walk, Brownian Motion, and Martingales读者反馈学科排名
|
|
|
发表于 2025-3-21 18:51:28
