| 书目名称 | International Symposium on Mathematics, Quantum Theory, and Cryptography | | 副标题 | Proceedings of MQC 2 | | 编辑 | Tsuyoshi Takagi,Masato Wakayama,Yasuhiko Ikematsu | | 视频video | | | 概述 | Includes selected papers by leading researchers.Presents the latest research on post-quantum cryptography.Enhances understanding of the mathematical background | | 丛书名称 | Mathematics for Industry | | 图书封面 |  | | 描述 | This open access book presents selected papers from International Symposium on Mathematics, Quantum Theory, and Cryptography (MQC), which was held on September 25-27, 2019 in Fukuoka, Japan. The international symposium MQC addresses the mathematics and quantum theory underlying secure modeling of the post quantum cryptography including e.g. mathematical study of the light-matter interaction models as well as quantum computing..The security of the most widely used RSA cryptosystem is based on the difficulty of factoring large integers. However, in 1994 Shor proposed a quantum polynomial time algorithm for factoring integers, and the RSA cryptosystem is no longer secure in the quantum computing model. This vulnerability has prompted research into post-quantum cryptography using alternative mathematical problems that are secure in the era of quantum computers. In this regard, the National Institute of Standards and Technology (NIST) began to standardizepost-quantum cryptography in 2016. .This book is suitable for postgraduate students in mathematics and computer science, as well as for experts in industry working on post-quantum cryptography.. | | 出版日期 | Conference proceedings‘‘‘‘‘‘‘‘ 2021 | | 关键词 | Cryptography for Quantum Computers; Post-quantum Cryptography; Quantum Computing; Number Theory; Represe | | 版次 | 1 | | doi | https://doi.org/10.1007/978-981-15-5191-8 | | isbn_softcover | 978-981-15-5193-2 | | isbn_ebook | 978-981-15-5191-8Series ISSN 2198-350X Series E-ISSN 2198-3518 | | issn_series | 2198-350X | | copyright | The Editor(s) (if applicable) and The Author(s) 2021 |
The information of publication is updating
书目名称International Symposium on Mathematics, Quantum Theory, and Cryptography影响因子(影响力)
书目名称International Symposium on Mathematics, Quantum Theory, and Cryptography影响因子(影响力)学科排名
书目名称International Symposium on Mathematics, Quantum Theory, and Cryptography网络公开度
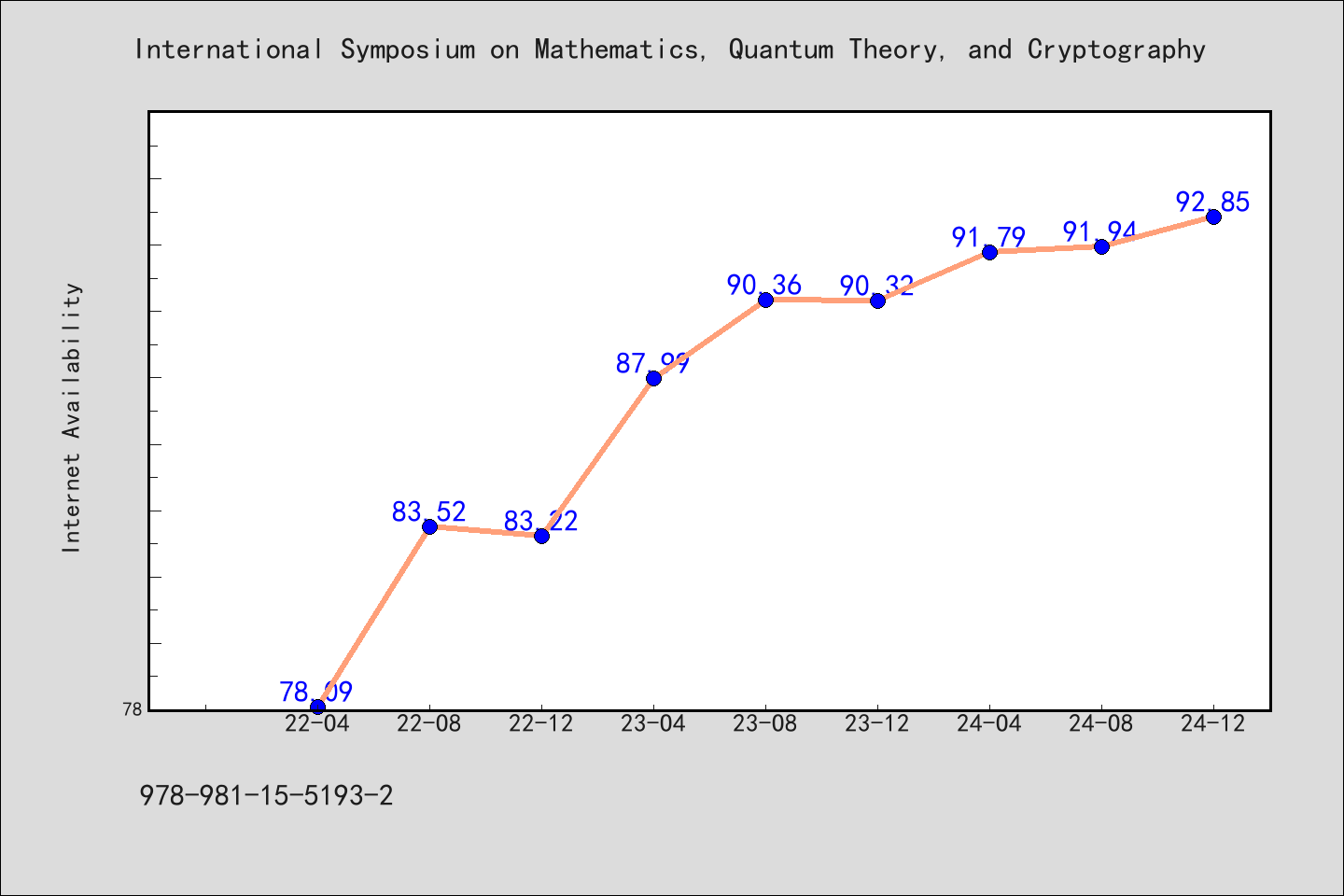
书目名称International Symposium on Mathematics, Quantum Theory, and Cryptography网络公开度学科排名
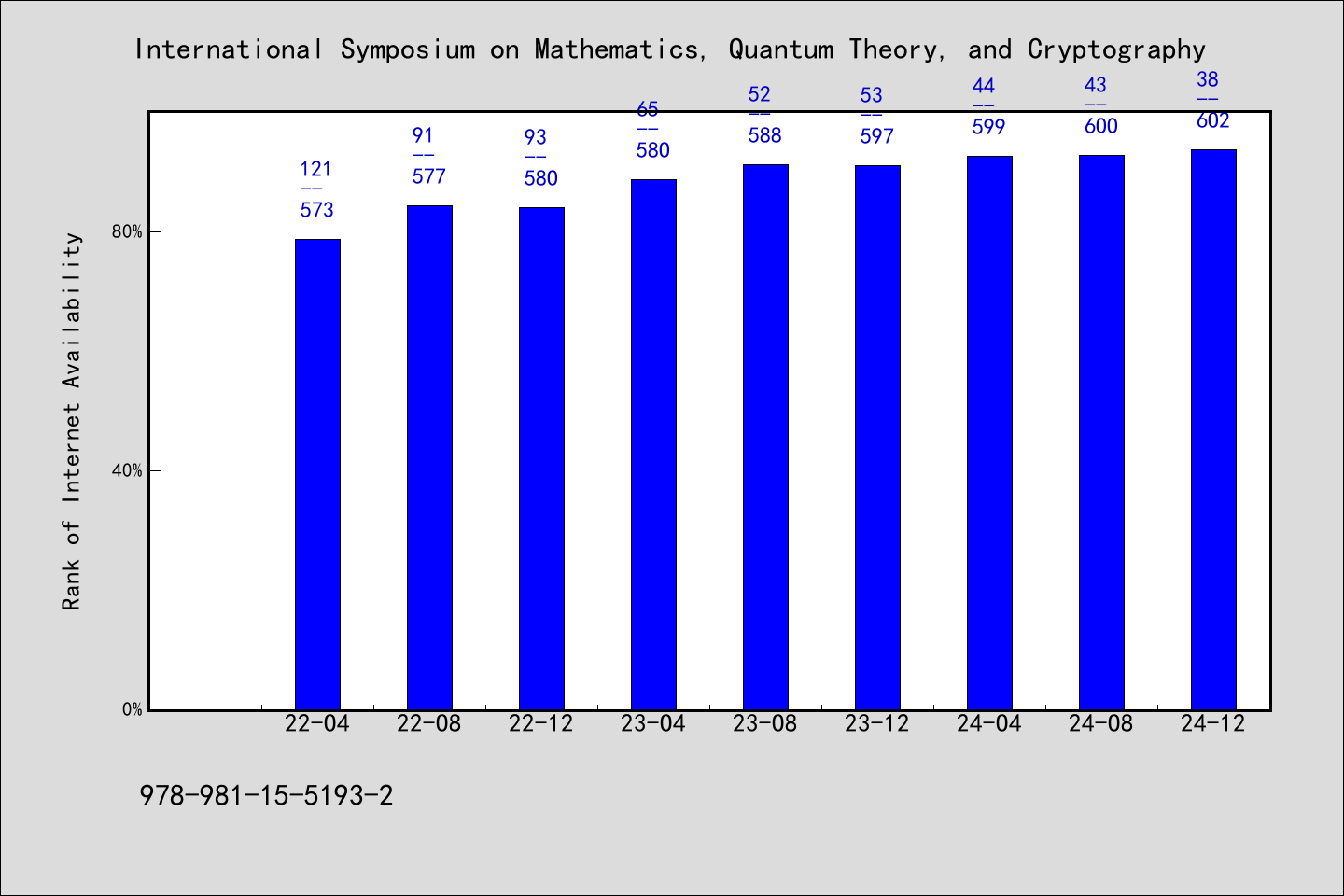
书目名称International Symposium on Mathematics, Quantum Theory, and Cryptography被引频次
书目名称International Symposium on Mathematics, Quantum Theory, and Cryptography被引频次学科排名
书目名称International Symposium on Mathematics, Quantum Theory, and Cryptography年度引用
书目名称International Symposium on Mathematics, Quantum Theory, and Cryptography年度引用学科排名
书目名称International Symposium on Mathematics, Quantum Theory, and Cryptography读者反馈
书目名称International Symposium on Mathematics, Quantum Theory, and Cryptography读者反馈学科排名
|
|
|
发表于 2025-3-21 18:34:02
