| 书目名称 | Harmonic Analysis on Symmetric Spaces—Higher Rank Spaces, Positive Definite Matrix Space and General | | 编辑 | Audrey Terras | | 视频video | | | 概述 | New edition extensively revised and updated.Includes many new figures and examples.New topics include random matrix theory and quantum chaos.Includes recent work on modular forms and their correspondi | | 图书封面 |  | | 描述 | .This text is an introduction to harmonic analysis on symmetric spaces, focusing on advanced topics such as higher rank spaces, positive definite matrix space and generalizations. It is intended for beginning graduate students in mathematics or researchers in physics or engineering. As with the introductory book entitled "Harmonic Analysis on Symmetric Spaces - Euclidean Space, the Sphere, and the Poincaré Upper Half Plane, the style is informal with an emphasis on motivation, concrete examples, history, and applications. The symmetric spaces considered here are quotients X=G/K, where G is a non-compact real Lie group, such as the general linear group GL(n,P) of all n x n non-singular real matrices, and K=O(n), the maximal compact subgroup of orthogonal matrices. Other examples are Siegel‘s upper half "plane" and the quaternionic upper half "plane". In the case of the general linear group, one can identify X with the space Pn of n x n positive definite symmetric matrices..Manycorrections and updates have been incorporated in this new edition. Updates include discussions of random matrix theory and quantum chaos, as well as recent research on modular forms and their corresponding L- | | 出版日期 | Textbook 2016Latest edition | | 关键词 | Eisenstein series; Harish-Chandra c-function; Helgason-Fourier transform; Poisson summation formula; Sel | | 版次 | 2 | | doi | https://doi.org/10.1007/978-1-4939-3408-9 | | isbn_softcover | 978-1-4939-8042-0 | | isbn_ebook | 978-1-4939-3408-9 | | copyright | Springer Science+Business Media New York 2016 |
The information of publication is updating
书目名称Harmonic Analysis on Symmetric Spaces—Higher Rank Spaces, Positive Definite Matrix Space and General影响因子(影响力)
书目名称Harmonic Analysis on Symmetric Spaces—Higher Rank Spaces, Positive Definite Matrix Space and General影响因子(影响力)学科排名
书目名称Harmonic Analysis on Symmetric Spaces—Higher Rank Spaces, Positive Definite Matrix Space and General网络公开度
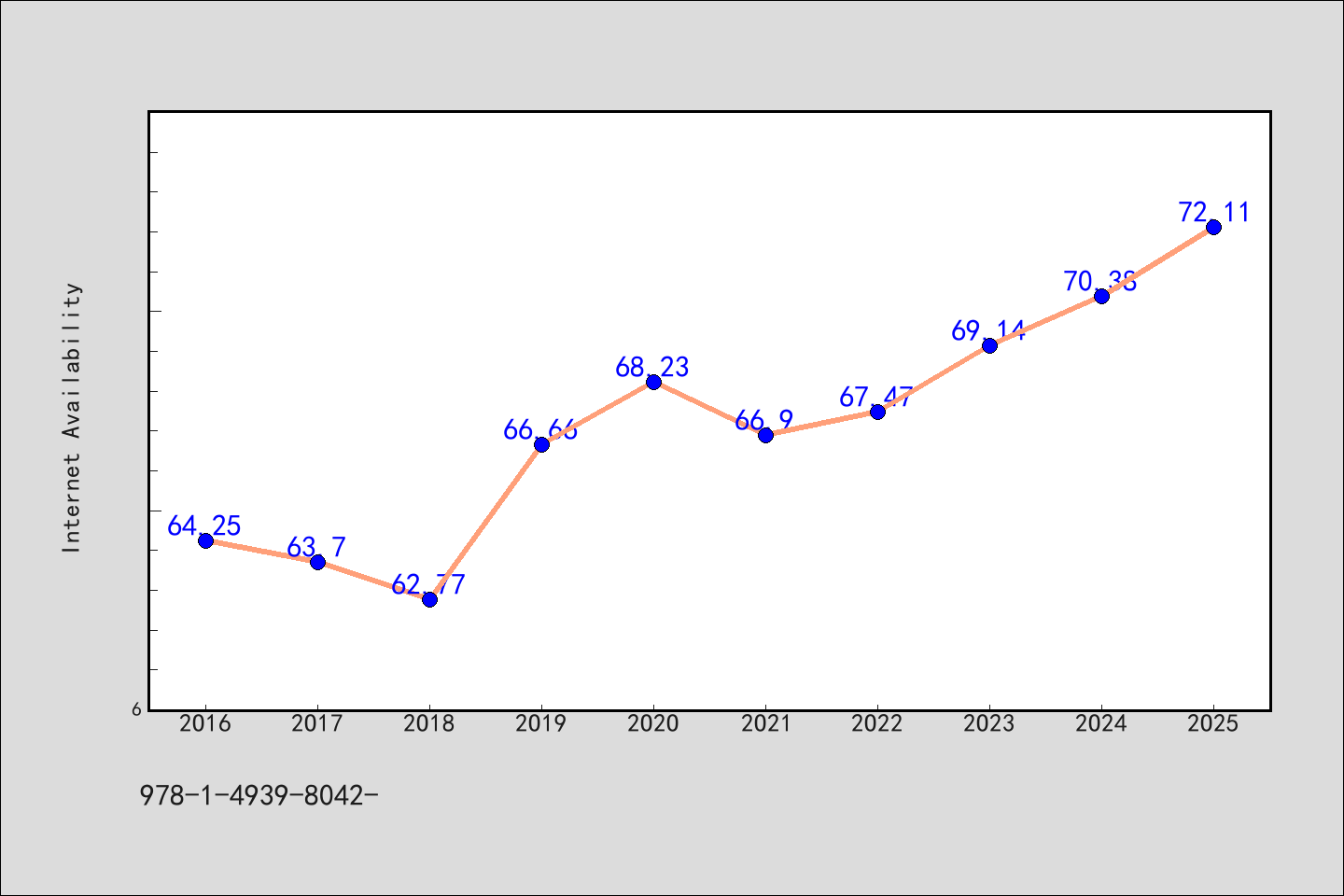
书目名称Harmonic Analysis on Symmetric Spaces—Higher Rank Spaces, Positive Definite Matrix Space and General网络公开度学科排名
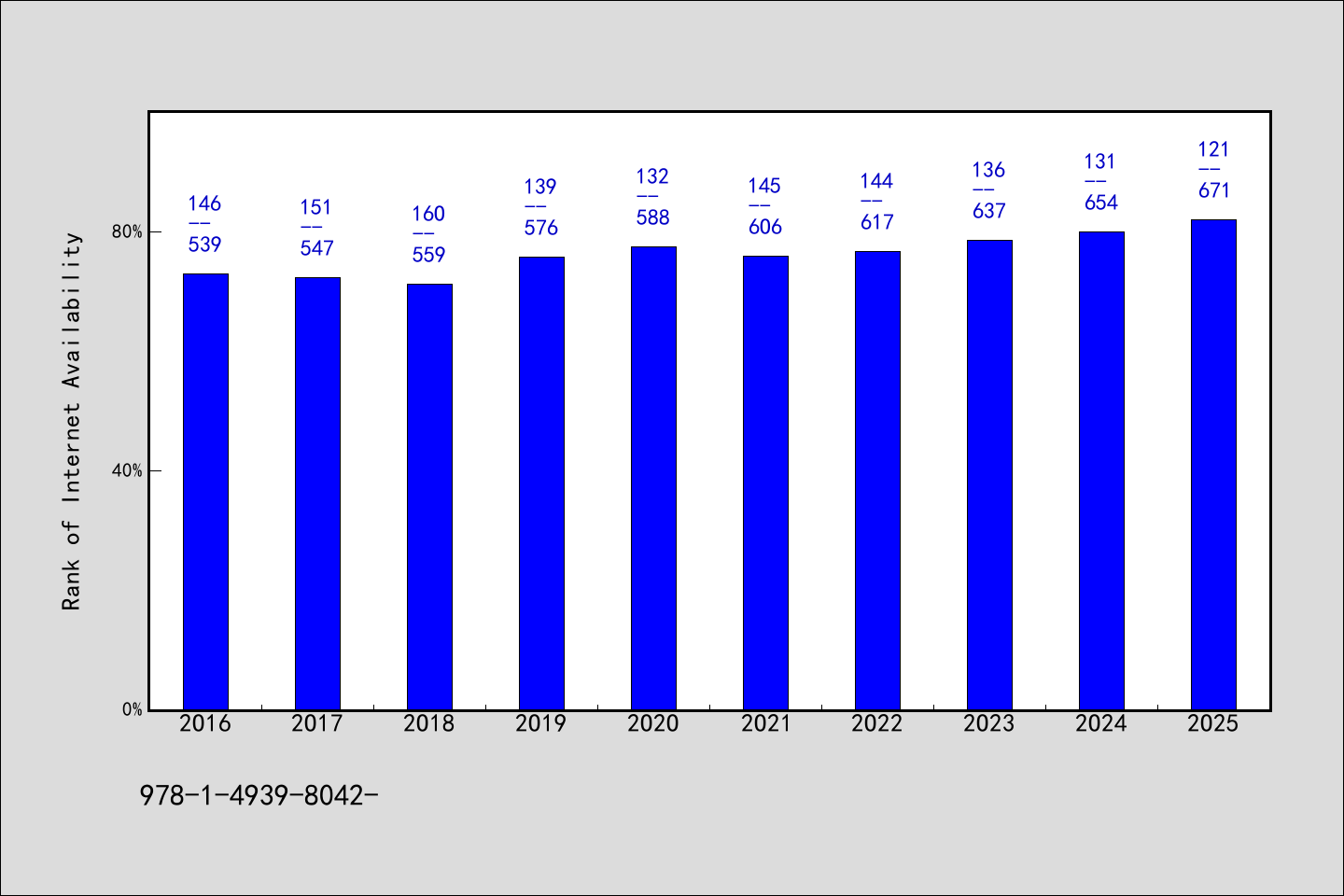
书目名称Harmonic Analysis on Symmetric Spaces—Higher Rank Spaces, Positive Definite Matrix Space and General被引频次
书目名称Harmonic Analysis on Symmetric Spaces—Higher Rank Spaces, Positive Definite Matrix Space and General被引频次学科排名
书目名称Harmonic Analysis on Symmetric Spaces—Higher Rank Spaces, Positive Definite Matrix Space and General年度引用
书目名称Harmonic Analysis on Symmetric Spaces—Higher Rank Spaces, Positive Definite Matrix Space and General年度引用学科排名
书目名称Harmonic Analysis on Symmetric Spaces—Higher Rank Spaces, Positive Definite Matrix Space and General读者反馈
书目名称Harmonic Analysis on Symmetric Spaces—Higher Rank Spaces, Positive Definite Matrix Space and General读者反馈学科排名
|
|
|
发表于 2025-3-21 16:07:44
