Titlebook: Elliptic Curves, Hilbert Modular Forms and Galois Deformations; Laurent Berger,Gebhard Böckle,John Voight Textbook 2013 Springer Basel 201

malcontented

书目名称Elliptic Curves, Hilbert Modular Forms and Galois Deformations影响因子(影响力)




书目名称Elliptic Curves, Hilbert Modular Forms and Galois Deformations影响因子(影响力)学科排名




书目名称Elliptic Curves, Hilbert Modular Forms and Galois Deformations网络公开度




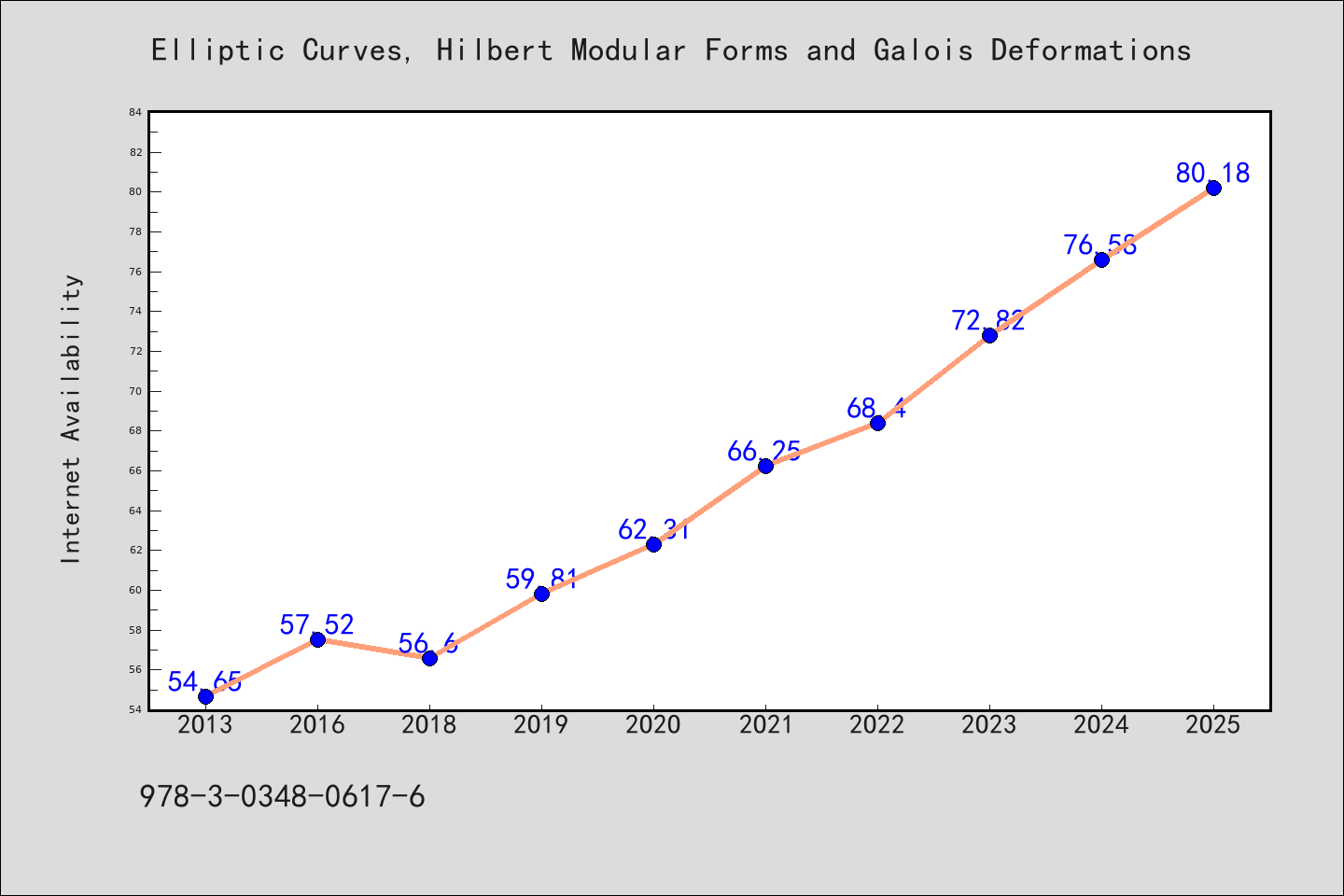
书目名称Elliptic Curves, Hilbert Modular Forms and Galois Deformations网络公开度学科排名




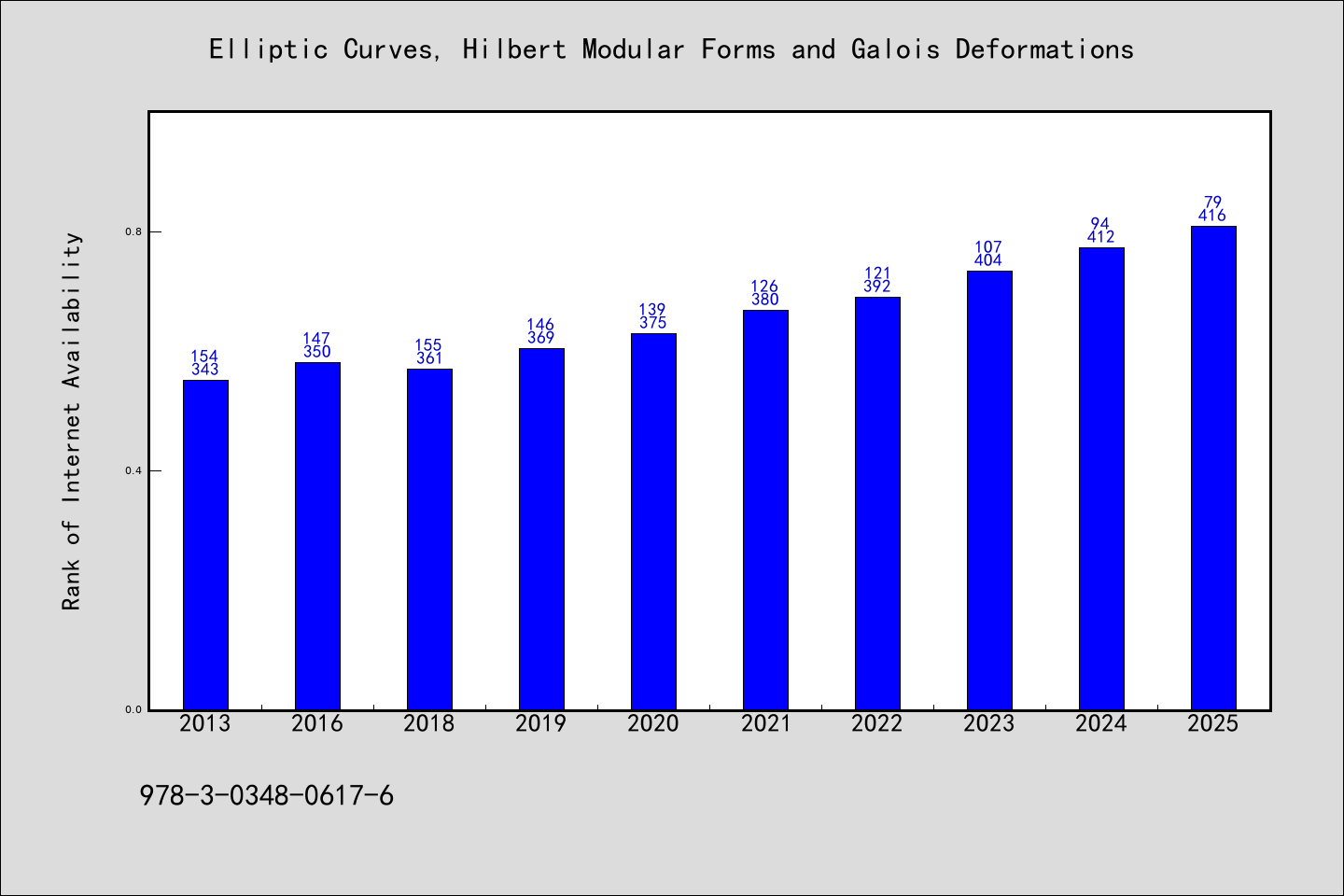
书目名称Elliptic Curves, Hilbert Modular Forms and Galois Deformations被引频次




书目名称Elliptic Curves, Hilbert Modular Forms and Galois Deformations被引频次学科排名




书目名称Elliptic Curves, Hilbert Modular Forms and Galois Deformations年度引用




书目名称Elliptic Curves, Hilbert Modular Forms and Galois Deformations年度引用学科排名




书目名称Elliptic Curves, Hilbert Modular Forms and Galois Deformations读者反馈




书目名称Elliptic Curves, Hilbert Modular Forms and Galois Deformations读者反馈学科排名

强壮

Arithmetic Aspects of Hilbert Modular Forms and Varietiesreplaced by a totally real number field. The aim of these notes is to present the basics of their arithmetic theory and to describe some of the recent results in the area. A special emphasis will be put on the following two subjects: images of Galois representations associated to Hilbert modular for

endoscopy

Explicit Methods for Hilbert Modular Formsa wide variety of mathematical problems. This subject has seen dramatic progress during the past half-century in an environment where both abstract theory and explicit computation have developed in parallel. Experiments will remain an essential tool in the years ahead, especially as we turn from cla

负担

Notes on the Parity Conjectureecture for elliptic curves over number fields. Along the way, we review local and global root numbers of elliptic curves and their classification, and we end by discussing some peculiar consequences of the parity conjecture.

腐蚀

https://doi.org/10.1007/978-3-322-91789-8a wide variety of mathematical problems. This subject has seen dramatic progress during the past half-century in an environment where both abstract theory and explicit computation have developed in parallel. Experiments will remain an essential tool in the years ahead, especially as we turn from classical contexts to less familiar terrain.

AVOW

Komplexe Zahlen und Funktionen,ecture for elliptic curves over number fields. Along the way, we review local and global root numbers of elliptic curves and their classification, and we end by discussing some peculiar consequences of the parity conjecture.

AVOW

热情赞扬

Notes on the Parity Conjectureecture for elliptic curves over number fields. Along the way, we review local and global root numbers of elliptic curves and their classification, and we end by discussing some peculiar consequences of the parity conjecture.

挥舞

strain

Laurent Berger,Gebhard Böckle,John VoightThe book contains the first published notes on the recent developments and major changes in Galois deformation theory during the last decade (deformations of pseudo-representations, framed deformation